New PhD student in the algebra group

We are happy to announce that Jonathan Tilling Niemann will start as a PhD student in the algebra group. The planned starting date is the 15th of March this year. Jonathan studied mathematics at KU and defended his master’s thesis today with great success obtaining the maximal grade. His PhD project will be supervised by Peter and Maria.

Jonathan will work within the area of maximal curves defined over a finite field. These are algebraic curves that attain the famous Hasse-Weil bound. Such curves are particularly interesting from the point of error-correcting codes as well. In recent years several new families of maximal curves have been found (among others a family discovered by Peter and Maria that is now called the Beelen-Montanucci family of maximal curves). Jonathan’s project is about finding common properties of these families and, if possible, to use the gained insights to find new families of maximal curves. The resulting impact in the area of error-correcting codes will be investigated as well.

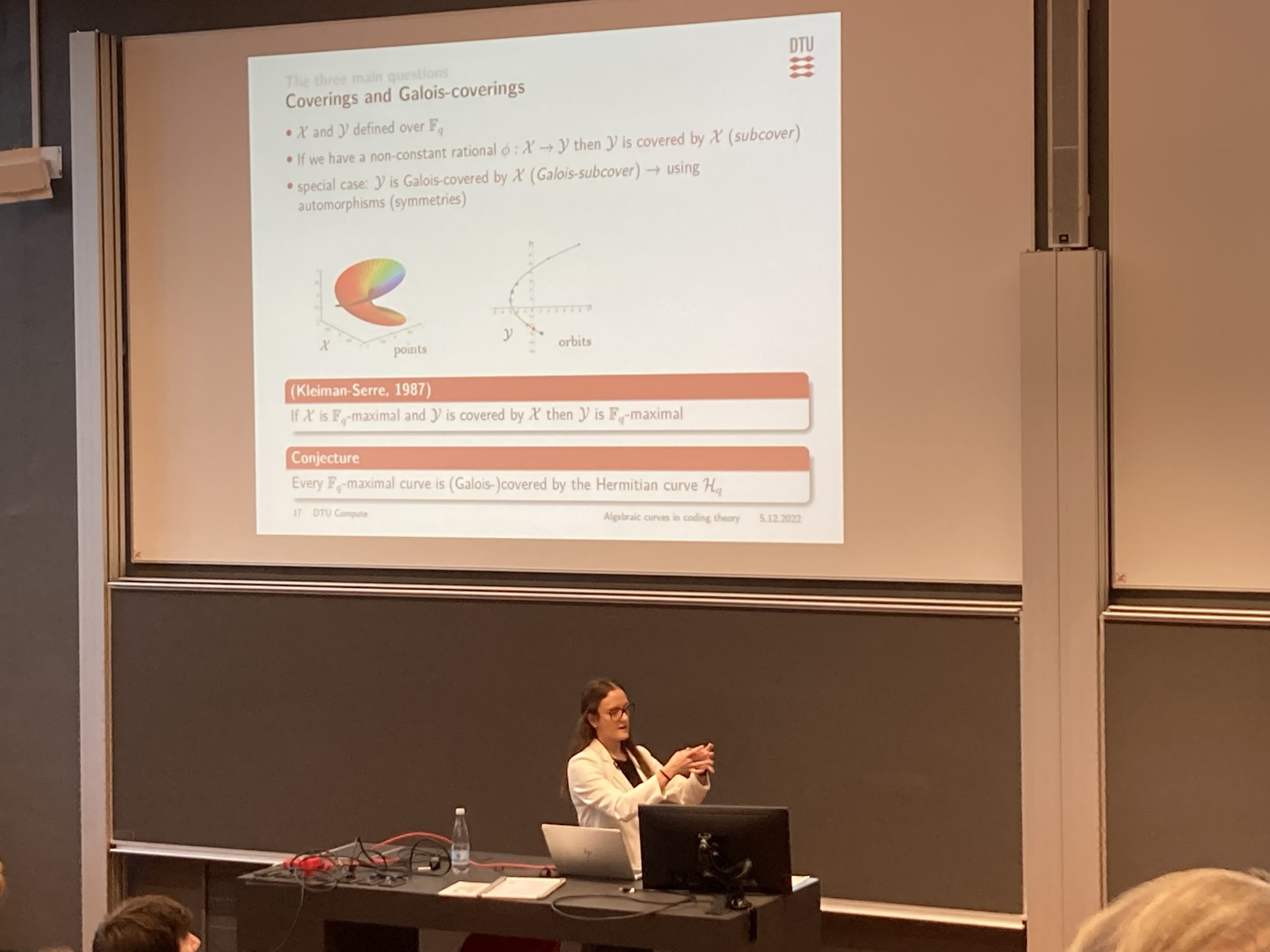
Today, Maria gave a talk for the department in connection to the seminar “Meet DTU Compute”. She talked about her work algebraic curves and their uses in coding theory. Title and abstract are given below.

Title: Algebraic curves in coding theory

Abstract: Whether colors in the rainbow or notes in a musical scale, there is a natural human desire to categorize objects, and classifying shapes by their geometric properties has always been a fundamental mathematical research area. Here shapes are grouped into basic geometric objects such as points, lines, figures. However, most geometrical objects occurring in science are so complex that they defy this traditional mathematical description making it hard to classify them. One solution is to use algebra to see what geometric objects have in common, which entails describing them as a set of equations. In this light, algebraic curves are the simplest objects one can study, as in the plane they are given by one single equation.

For a long time, the study of algebraic curves was the province of pure mathematicians. But then, in a series of three papers in the period 1977-1982, Goppa found important applications of algebraic curves over finite fields, and especially of those having many rational points, to coding theory (reliable communication). Since then, many other applications followed naturally.

In this presentation, after a general introduction to coding theory and algebraic curves, the main ideas behind Goppa’s construction will be described together with some more recent applications of algebraic curves in Information Theory.

Today Ph.D. student Lara Vicino has given an online talk as part of the series of on-line seminars: Galois Geometries and their applications – Young seminars (organized by Department of Mathematics and Physics – Università degli Studi della Campania “Luigi Vanvitelli”). She talked about recent results on Weierstrass semigroups at points of a maximal curve with the third largest genus. Such curves are of particular interest in coding theory since they give rise to excellent AG codes and fast decoders are known for such codes. The work is joint with Peter Beelen and Maria Montanucci.

This and next week, the algebra group will host Vincent Neiger as a visitor. He previously was a postdoc in the algebra group, but now is an Associate Professor (Maître de Conférences) at Sorbonne University, Faculty of Science and Engineering. Vincent is an expert in computer algebra and has put his name to various very fast algorithms. We look forward to working with him!

Each year the Ph.D. school of DTU Compute organizes a Ph.D. bazar, where among other things Ph.D. students can present their work. Ph.D. student Lara Vicino presented her work with her supervisors on the Weierstrass semigroups of maximal curves and illustrated the use of numerical semigroups in general. Here you can see a picture of her in action today.

In the period 13th June till 3rd July, the algebra group will be visited by Prof. Sudhir Ghorpade (IIT Bombay) and Assistant Prof. Mrinmoy Datta (IIT Hyderabad). The goal is to continue the study of a famous class of algebraic geometry codes, the projective Reed-Muller codes.

Yesterday, Leonardo Landi obtained his Ph.D. degree! The defense of his Ph.D. thesis “Semigroups, curves and AG codes” started with him giving an interesting talk about his work. After that, he answered in a very convincing way all questions posed by the members of his Ph.D. committee.

We would like to congratulate Leonardo and wish him good luck with his future career!

A preprint on fast decoding of AG codes is now available on arXiv here. It was written by Peter Beelen, Johan Rosenkilde, and Grigory Solomatov. In the preprint, it is explained how to decode any AG code fast. In fact, the obtained decoding complexity is at least as fast as any previously known algorithm even when specified to particular AG codes such as the highly studied one-point Hermitian codes. It should be said however, that for Reed-Solomon codes, which can be viewed as AG codes as well, there exists a slightly faster decoding algorithm.

The result in the preprint may well become the bench mark for any future work on decoding AG codes. They also appeared as a chapter in the recent PhD thesis of Grigory Solomatov. Needless to say that we are very excited by the obtained results!

Update (November 2022): the paper has appeared in the highly esteemed journal IEEE Transactions on Information Theory!

The article  “On the constant D(q) defined by Homma” by the algebra group members Peter Beelen, Maria Montanucci, and Lara Vicino has been accepted for publication in the Proceedings of the 18th Conference on Arithmetic, Geometry, Cryptography, and Coding Theory in the AMS book series Contemporary Mathematics (CONM). In this article, we investigate the asymptotic behaviour of the number of points on an algebraic curve defined over a finite field in relation to its degree. A preprint of this article can be found here.

Today, Grigory defended his Ph.D. thesis successfully! After giving an excellent presentation on his work, he answered various questions from his opponents. During his Ph.D. Grigory obtained various results on computational aspects of algebraic geometry (AG) codes, including results on encoding and decoding such codes. The results include decoding any AG code very efficiently, matching and very often improving preexisting decoders.

We would like to acknowledge the support from The Danish Council for Independent Research (DFF-FNU) for the project Correcting on a Curve, Grant No. 8021-00030B. Their support made Grigory’s Ph.D. project and the obtained results a reality.

Congratulations to Grigory with obtaining his Ph.D.-degree!